
Today probably there are two different very popular ancient traditional methods are available for school students to conquer mathematics. With either of these techniques students are able to do large and complex calculations in their head without need of any modern day electronic devices like calculators etc. These techniques are: 1) Abacus Math and 2) Vedic Math. Before we indulge straight into the differences let us first know what these two methods are.
The abacus is an ancient tool invented during Greek and Roman times. Over the period abacus has evolved into different types as it traveled across the world. However it was in China the abacus was innovated further and used heavily in day-to-day life for calculations. Abacus math is a technique derived from this very old technique and fits right into the modern day world. Abacus use can build confidence, provide a sense of achievement, promote intuitive thinking, enhance problem-solving capability, stimulate creativity and improve concentration and mental endurance.
Vedic Mathematics has no legal definition. Hindu philosophy is based on Vedas and mathematics translated from these texts is termed as Vedic Mathematics. These ancient scriptures were rediscovered by Sri Bharati Krsna Tirthaji (1884-1960) and he translated the complex text into simpler understandable mathematics. The system is based on 16 Vedic sutras or aphorisms, which are actually word-formulae describing natural ways of solving a whole range of mathematical problems. These 16 one-line formulae originally written in Sanskrit, which can be easily memorized, enables one to solve long mathematical problems quickly. These 16 Jaw-Dropping Sutras or Word Formulae is easy to implement in schools – a reason behind its enormous popularity among academicians and students. It complements the Mathematics curriculum conventionally taught in schools by acting as a powerful checking tool and goes to save precious time in examinations. These formulae describe the way the mind naturally works and are therefore a great help in directing the student to the appropriate method of solution. They are called “Vedic” because the sutras are said to be contained in the Atharva Veda – a branch of mathematics and engineering in the Ancient Indian Scriptures.
With Abacus math, abacus is used as a tool to learn calculations. Beads are moved up and down and various columns to represent the number. When children use both hands to move the abacus beads to perform arithmetic calculations, there is quick communication between the hands and the brain that stimulates both the right and left hemispheres of the brain. This promotes rapid, balanced whole brain development. However it is believed that Abacus math should be started at very early childhood, as young as age 4. Abacus math starts at very basic level by teaching the numbers and then progressing to calculations. Eventually the child retains the memory of bead positions and the relevant notation. Abacus math if started during later ages can create a bit of hindrance.
Vedic Mathematics is entirely doable in mind alone. Vedic Mathematics also starts at a basic level of numbers and gradually progressing to simple additions, subtractions, multiplications and division. Vedic Mathematics goes much more beyond just the basic calculations. With Vedic Mathematics one can also solve complex geometrical theorems, algebraic problems, Calculus etc. Vedic Mathematics can be started at later ages as well without any difficulty. The key in both techniques is to practice and implement the methods in day-to-day life.
The Vedic Mathematics system uses a collection of sixteen sutras and some sub-sutras. Perhaps the most striking feature of the Vedic system is its coherence. Instead of a hotch-potch of unrelated techniques the whole system is beautifully interrelated and unified: the general multiplication method, for example, is easily reversed to allow one-line divisions and the simple squaring method can be reversed to give one-line square roots. And these are all easily understood. This unifying quality is very satisfying; it makes mathematics easy and enjoyable and encourages innovation. In the Vedic system 'difficult' problems or huge sums can often be solved immediately by the Vedic method. These striking and beautiful methods are just a part of a complete system of mathematics which is far more systematic than the modern 'system'. Vedic Mathematics manifests the coherent and unified structure of mathematics and the methods are complementary, direct and easy.
According to some learned scholars, the main criticism of Vedic Mathematics is that the Sutras have not been located in the extant main stream Vedic literature popularly known and therefore it is not precise to give the name Vedic Mathematics. Since the Vedic literature is very extensive, with many documents not having been recorded, it is still quite possible that the Sutras could be located in some texts. Some Vedic texts have also been lost in floods, other natural calamities etc. However in deed the word 'Vedic' refers not only to actual texts but also has a literal meaning of 'knowledge'. It does not make any difference to the potency or application of Vedic Mathematics whether the Sutras are located in ancient texts or not or for that matter the validity of the name is not a significant issue in respect to benefit of learners are concerned at least.
In one of Aesop's fables a bird was trying to drink from a jug but could not reach down to the level of the water. After a while instead of struggling to get his head down far enough the bird went and got some stones which he dropped into the jug until the level had raised enough for him to drink the water. For example, 'Vertically and Crosswise` is one of these Sutras. These formulae describe the way the mind naturally works and are therefore a great help in directing the student to the appropriate method of solution. Some of the sutras seem to fit neatly with a well-known mental function. For example, Transpose and Apply would describe the reversing that we often use in our thinking.
Now take an example general in nature for two digit multiplication.
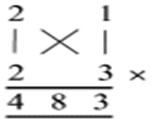
Multiply vertically on the left: 2 x 2 = 4. This gives the first figure of the answer. Multiply crosswise and add: 2 x 3 + 1 x 2 = 8. This gives the middle figure. Multiply vertically on the right: 1 x 3 = 3. This gives the last figure of the answer.
Now try 32 x 38 = 1216. Both numbers here start with 3 & the last figures add up to 10 (8+2=10). So we just multiply 3 by 4 (the next number up) to get 12 for the first part of the answer & we multiply the last figures to get the last part of the answer. Diagrammatically:
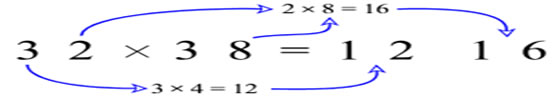
With the similar logic if we multiply 94 with 96, we just multiply 9 by 10 (the next number up) to get 90 for the first part of the answer & we multiply the last figures to get the last part of the answer. Hence 94X96=9024 (9X10=90 is the first part and 4X6=24 is the last part). Similarly try 81 x 89 = 7209, 8 X (8+1) = 72 then we put 09 since we need two figures because the base is 10². Using the same logic 99 X 91 = 9009. Now multiplying numbers just over 100. 103 x 104 = 10712. The answer is in two parts: 107 & 12, 107 is just 103 + 4 (or 104 + 3), & 12 is just 3 x 4. Similarly 107 x 106 = 11342, 107 + 6 (or 106 + 7) = 113 and 7 x 6 = 42.
Schools all over the world utilize pure mathematics for their curriculum. Therefore, an in-depth knowledge of pure mathematics is essential from the examination point of view. Unfortunately, the mathematics that is taught in most schools and colleges lack flexibility &, therefore, becomes 'boring' to the students. Fixed methods are also used for all operations thereby making the process of calculation lengthy and tiresome, resulting in making mathematics a nightmare before exams.
But please note from the above illustrations. We are using different procedure to compute the sums i.e. multiplications and the particular procedure taken to compute the sum is primarily decided upon the characteristics of the number or pattern. We will certainly adopt the method that suits best but that is definitely not meaning that other alternative approaches are not applicable. So you have lot of games to play with Vedic Mathematics. It also deals with non-linear methods of developing pattern-recognition tremendously helping a student in competitive & entrance exams later in life. Hence Vedic Mathematics is not an additional burden for students, teachers & parents, but complements the existing mathematics syllabus and makes mathematics far more interesting and enjoyable for all, especially those who shy away from mathematics.
Sitangshu Ghoshal
(Readers may contact the author for any further clarifications if necessary through email [email protected])




 Avoid pre-registered SIMs, buyer and seller liable for prosecution – Ursula Owus...
Avoid pre-registered SIMs, buyer and seller liable for prosecution – Ursula Owus...
 Election 2024: Mahama has nothing new to offer Ghanaians, Bawumia is the future ...
Election 2024: Mahama has nothing new to offer Ghanaians, Bawumia is the future ...
 OSP files fresh charges against ex- PPA Boss
OSP files fresh charges against ex- PPA Boss
 Withdraw unreasonable GH¢5.8m fine against former board members – ECG tells PURC
Withdraw unreasonable GH¢5.8m fine against former board members – ECG tells PURC
 Akroma mine attack: Over 20 armed robbers injure workers, steal gold at Esaase
Akroma mine attack: Over 20 armed robbers injure workers, steal gold at Esaase
 Those who understand me have embraced hope for the future — Cheddar
Those who understand me have embraced hope for the future — Cheddar
 Ghana will make maiden voyage into space should Bawumia become President — Chair...
Ghana will make maiden voyage into space should Bawumia become President — Chair...
 Train crash: Despite the sabotage, we shall not be deterred and will persevere —...
Train crash: Despite the sabotage, we shall not be deterred and will persevere —...
 Tema-Mpakadan railway project a perversion of the original viable concept design...
Tema-Mpakadan railway project a perversion of the original viable concept design...
 Train crash: Elsewhere, everyone involved in the test will either be fired or re...
Train crash: Elsewhere, everyone involved in the test will either be fired or re...
